7.1 Integration of Parts
You should remember that the product rule is :
By rearranging this equation you see that:
By using the substitution rule and replacing u for f(x), v for g(x), du for f'(x)dx, and dv for g'(x)dx, you will see that the formula for integreation of parts becomes:


Let's look at a couple of examples!
1) 
Choose your simplest component for u. In this case that would be x. Make a table of your u, du, v, and dv.

Choose your simplest component for u. In this case that would be x. Make a table of your u, du, v, and dv.
Now try a problem!
Now plug this equation back into the end of the first integration of parts:
Here is a good site for Integration of Parts:
http://www.sosmath.com/calculus/integration/byparts/byparts.html
http://www.sosmath.com/calculus/integration/byparts/byparts.html
Saladang Song: My obsession with food has led me to this great Thai restaurant. I highly recommend it (especially the Yellow Curry) and it's very well priced.
363 S Fair Oaks Ave (Cross Street: W Bellevue Drive)Pasadena, CA 91105
363 S Fair Oaks Ave (Cross Street: W Bellevue Drive)Pasadena, CA 91105
Kyle, you're up next!










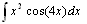











0 Comments:
Post a Comment
<< Home