Section 6.1: Areas Between Curves

Yay it's my turn now. As I look at these problems more and more, they seem less scary, so if any of you are worried about this concept, as I was, don't worry because with some practice, I'm sure that you can kick these problems' butts! Before I get into summarizing this lesson, here is a link for any further information: http://tutorial.math.lamar.edu/AllBrowsers/2413/AreaBetweenCurves.asp

Ok so anywho, this lesson takes integrals as we have learned them a step further. Now, instead of finding the area beneath a curve on a given domain, we have to find the area between two different curves on a given domain. It's just a little extra wrinkle that we have to deal with, but no big deal. Let's say that we are given the following graph:



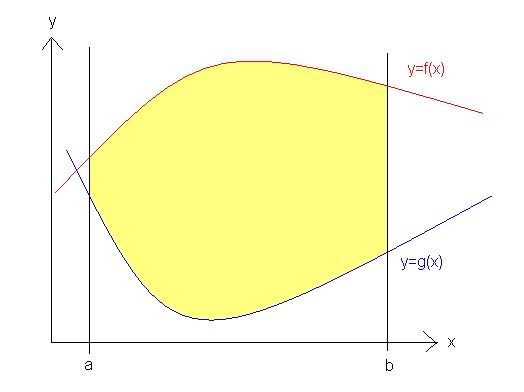
The red curve represents the upper curve, y=f(x), and the blue curve represents the lower curve, y=g(x). a and b represent the given domain, and the shaded yellow area represents what you are trying to find when you solve one of these problems. In order to find this area, you can use the following general formula:




Now we can use that for a fun sample problem!! Brace yourselves...



First, we can draw the graph of this situation. The yellow graph is the area that we are trying to find.



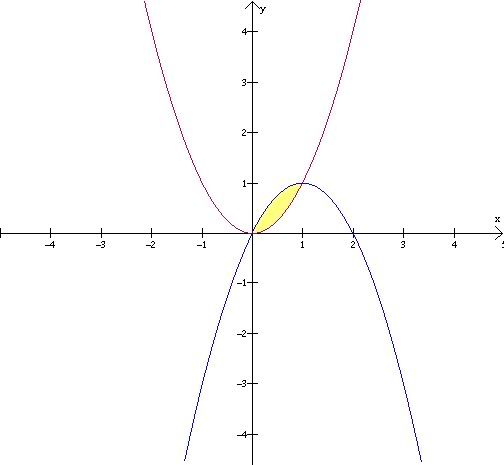
From this picture, we can see that the upper boundary is the blue curve, or y=2x-x(x), and the lower boundary is y=x(x). Therefore, the area is gonna be (upper boundary-lower boundary)dx or (2x-x(x)-x(x)). Also, the region is between x=0 and x=1. Therefore, the total area is



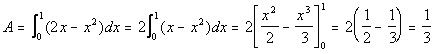
So, your answer is 1/3. YAY JESSICA YOU'RE NEXT HAVE FUN!!