Section 9.2: Direction Fields and Euler's Method

Hey all...so today's lesson deals with sketching and approximation to figure out the shape of the curve of a function without any real in-depth calculations. According to the book, the definition for a Direction (or Slope) Field is: "If we draw short line segments with the slope F(x,y) at several points (x,y), the result is called a direction field (or slope field)." In other words, you are given an equation set equal to a derivative. For example, you could be given y'=x+xy. Then, you make a table of values divided into three columns: x, y, and y'. Pick a random pair of points for your (x,y) coordinate, plug this pair into your equation, and figure out the slope of the function at that particular point. Draw a short line at the designated point that has approximately the same slope as the slope that you just found. Here is an example:

Sketch the direction field for the differential equation y'=(x+xy)-y.

First, set up a table of values.

x y y'
-1 -1 -1
0 0 0
1 1 1
etc.

Now, draw in the slopes of the function at the given points. The end result should look like:



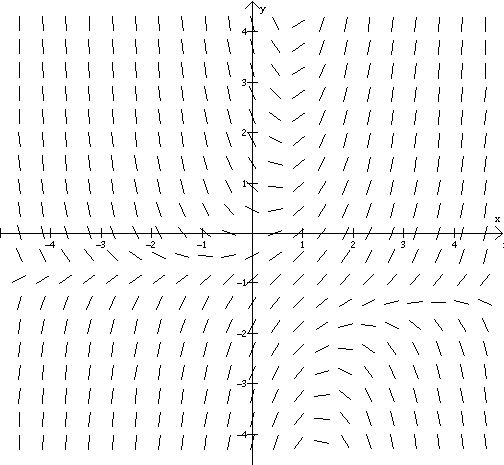
Of course, this is a very inexact method. Euler's method seeks to make the process of drawing a direction field a little more accurate. "For the general first-order initial-value problem y'=F(x,y), y(x0)=y0, our aim is to find approximate values for the solution at equally spaced numbers x0, x1=x0+h, x2=x1+h, ..., where h is the step size. The differential equation tells us that the slope at (x0, y0) is y'=F(x0, y0. The general equation to express Euler's rule is:

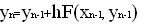
Now, let's apply this concept to a real problem.

Use Euler's method with step size .1 to construct a table of approximate values for the solution of the initial-value problem. y'=x+y, y(0)=1

y1=1+.1(0+1)=1.1
y2=1.1+.1(.1+1.1)=1.22
etc.

So as you can see, Euler's method allows us to draw a much more exact direction field by giving us more exact values for slopes. Here's a fun link for your personal enjoyment: http://tutorial.math.lamar.edu/AllBrowsers/3401/DirectionFields.asp

BRIAN YOU ARE NEXT HAVE FUN!

Two mathematicians were having dinner in a restaurant, arguing about the average mathematical knowledge of the American public. One mathematician claimed that this average was woefully inadequate, the other maintained that it was surprisingly high.
"I'll tell you what," said the cynic. "Ask that waitress a simple math question. If she gets it right, I'll pick up dinner. If not, you do."
He then excused himself to visit the men's room, and the other called the waitress over.
"When my friend returns," he told her, "I'm going to ask you a question, and I want you to respond 'one third x cubed.' There's twenty bucks in it for you." She agreed.
The cynic returned from the bathroom and called the waitress over. "The food was wonderful, thank you," the mathematician started. "Incidentally, do you know what the integral of x squared is?"
The waitress looked pensive, almost pained. She looked around the room, at her feet, made gurgling noises, and finally said, "Um, one third x cubed?"
So the cynic paid the check. The waitress wheeled around, walked a few paces away, looked back at the two men, and muttered under her breath, "...plus a constant."